Understanding the concept of decimals and fractions is essential in mathematics. Decimals are a way of representing numbers that are not whole numbers. In contrast, fractions represent parts of a whole or a group.

In this article, we will explore decimals and fractions and learn how to convert decimals to fractions step by step.

What is a Decimal?

A decimal is a way of representing a number that is not a whole number. It is a way of expressing fractions using the base 10 numbering system. A decimal number consists of two parts: a whole number part and a fractional part, separated by a decimal point.

The whole number part follows the same place value system as whole numbers, while the fractional part represents the parts of a whole that are smaller than one.

For example, the decimal number 3.14 can be considered 3 whole units plus 0.14 of another unit. After the decimal point, the first digit represents tenths, the second digit represents hundredths, and so on. In this case, 0.14 represents 14 hundredths.

What is a Fraction?

A fraction is a way of representing a part of a whole or a group of items. It consists of two parts: the numerator, which is the number above the line, and the denominator, which is the number below the line.

The numerator represents the number of equal parts that are being considered. In contrast, the denominator represents the total number of equal parts in the whole or group.

For example, if we have a pizza that is divided into 8 equal slices, and we eat 3 of those slices, we can represent this using the fraction 3/8. The numerator, 3, represents the number of slices we ate. In contrast, the denominator, 8, represents the total number of slices in the pizza.

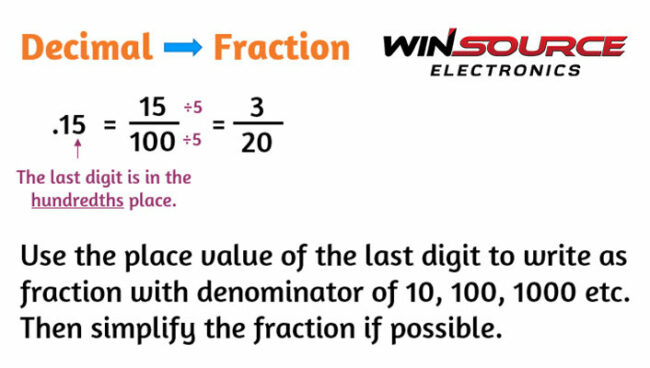
How to Convert a Decimal to a Fraction?

Converting a decimal to a fraction involves the following steps:

Step 1

Write the decimal number as the numerator of a fraction, with 1 as the denominator. For example, if the decimal number is 0.75, the fraction would be 0.75/1.

Step 2

To remove the decimal point, multiply both the numerator and denominator by a power of 10. The power of 10 should be equal to the number of decimal places in the decimal number.

For example, if the decimal number has two decimal places, multiply both the numerator and denominator by 100 (10^2). In our example, this would give us (0.75 x 100)/(1 x 100) = 75/100.

Step 3

Simplify the fraction by dividing both the numerator and denominator by their greatest common factor (GCF).

In our example, the GCF of 75 and 100 is 25, so we can simplify the fraction to (75 ÷ 25)/(100 ÷ 25) = 3/4.

Step4

If necessary, convert the simplified fraction into a mixed number by dividing the numerator by the denominator and writing the result as a whole number followed by a fraction.

For example, if we had simplified our fraction to 7/4, we could convert it into a mixed number by dividing 7 by 4 to get 1 with a remainder of 3. The mixed number would then be written as 1 3/4.

Conclusion

Understanding decimal fraction conversion is essential for various mathematical calculations and real-life applications.

Whether you’re dealing with financial figures, measurements, or ratios, converting decimals to fractions allows for greater flexibility and accuracy in your calculations. Hope that covered what you were looking for. If not, get in touch with us here at WIN SOURCE to have any of your queries answered.