Decimals and fractions are fundamental concepts in mathematics, often encountered in everyday life, from measuring ingredients in a recipe to calculating distances on a map.
Converting a decimal to a fraction might seem daunting at first, but with a clear understanding and simple step-by-step approach, anyone can master this skill.
This article by WIN SOURCE aims to break down the process of how to convert a decimal to fraction, providing practical examples and a conversion table to make the learning experience even more accessible.
Table of Contents
ToggleWhat is a Decimal?
A decimal is a way of representing parts of a whole number that fall between whole numbers. It consists of two main parts: the whole number part and the decimal part, separated by a dot or period. For instance, in the decimal 3.75, the whole number is 3, and the decimal part is 75.
What is a Fraction?
A fraction represents a part of a whole, typically consisting of two numbers separated by a line (fraction bar). The number above the line is called the numerator, representing how many parts we have, and the number below the line is called the denominator, indicating the total number of equal parts the whole is divided into. For example, in the fraction 3/4, 3 is the numerator, and 4 is the denominator.
How to Convert a Decimal to Fraction
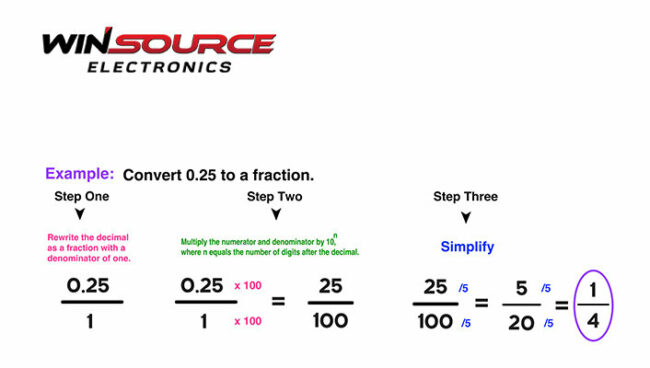
Converting a decimal to a fraction involves translating the decimal value into a fraction with a numerator and denominator. Follow these steps to accomplish this conversion:
Step 1: Identify the Decimal
Begin by identifying the decimal value you want to convert. For example, consider the decimal 0.625.
Step 2: Count Decimal Places
Count the number of decimal places in the given decimal. In our example, there are three decimal places in 0.625.
Step 3: Create the Fraction Denominator
Create a denominator for the fraction by using the number 1 followed by as many zeros as there are decimal places. For our example, the denominator would be 1000.
Step 4: Convert the Decimal to a Fraction
To convert the decimal to a fraction, write the decimal value as the numerator and the denominator you just created. In our example, the fraction becomes 625/1000.
Step 5: Simplify the Fraction
If possible, simplify the fraction by finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it. In our case, both 625 and 1000 can be divided by 125, resulting in the simplified fraction 5/8.
Where are Decimals used in Real Life?
Decimals, often encountered in everyday scenarios, play a crucial role in a multitude of real-life situations. Understanding their significance and being able to convert them to fractions can empower individuals to make informed decisions and tackle practical challenges.
Here are some common areas where decimals are used in real life:
1. Measurement and Quantities:
Decimals are the go-to representation when dealing with measurements. Whether it’s gauging temperature or measuring ingredients for a recipe, decimals allow for precise and accurate calculations. For instance, converting 2.5 meters to 250 centimeters involves a decimal-to-fraction conversion (2.5 meters = 250/100 cm).
2. Financial Transactions:
In the realm of finance, decimals are ubiquitous. From calculating interest rates on loans and investments to managing personal budgets, decimals ensure accuracy in computations. When determining a sales tax of 6.75% on a $100 purchase, understanding decimals is essential (6.75% = 0.0675).
3. Scientific Notation:
Decimals find extensive use in scientific notation, a way to represent very large or very small numbers efficiently. Converting numbers to scientific notation often involves transforming decimals into fractions of powers of ten. For instance, the speed of light, approximately 299,792,458 meters per second, can be expressed as 2.99792458 × 10^8 m/s.
4. Medicine and Healthcare:
In medical contexts, decimals aid in accurate dosing of medications and measurements of vital signs. Converting decimal dosages into fractions helps ensure the right amount of medication is administered. For example, converting a dosage of 1.25 mg to a fraction (1.25 mg = 5/4 mg) ensures precise administration.
5. Engineering and Construction:
Engineers and architects frequently work with decimals to create detailed plans and designs. Converting decimals to fractions allows for precise measurements when constructing buildings, bridges, or other structures.
6. Probability and Statistics:
Decimals are fundamental in probability and statistics, facilitating the representation of probabilities and percentages. When calculating the probability of drawing a spade from a standard deck of cards (13 spades out of 52 cards), converting decimals to fractions helps simplify the calculation (13/52).
7. Currency Exchange:
When traveling internationally or engaging in global trade, decimals are essential for currency exchange rates. Converting different currency values involves understanding decimals and performing conversions, such as converting 1.5 USD to Euros.
8. Technology and Computing:
Decimals are integral to computer programming and data representation. Converting decimals to binary or hexadecimal forms is crucial for encoding and transmitting data in various digital systems.
9. Sports and Recreation:
In sports, decimals come into play when analyzing statistics or determining scores. Converting a baseball player’s batting average of 0.325 to a fraction (325/1000) provides insights into their performance.
Generally, decimals are far from abstract concepts confined to mathematics classrooms. They permeate various aspects of our lives, enabling us to make informed decisions, carry out precise measurements, and engage with the world around us. By mastering the skill of converting decimals to fractions, individuals can enhance their numerical literacy and confidently tackle real-life challenges across diverse fields.
The Real-Life Utility of Fractions
Fractions, often seen as fundamental components of mathematics, play an integral role in countless real-life scenarios. Understanding their practical applications and being able to work with fractions empowers individuals to navigate everyday challenges and make informed decisions. Here’s a glimpse into the various areas where fractions are prominently used:
1. Cooking and Baking:
Fractions are essential in the culinary world, where precise measurements are crucial. Recipes often call for fractions of ingredients, such as 1/2 cup of flour or 3/4 teaspoon of salt. Accurate measurements ensure the desired taste and texture of dishes.
2. Construction and Carpentry:
In construction, fractions are employed to measure and cut materials accurately. Carpenters use fractions to determine dimensions, angles, and proportions for building structures and crafting furniture.
3. Home Improvement:
Whether you’re repainting a room or laying tiles, fractions are invaluable for estimating quantities of materials needed. Calculating how many tiles are required for a specific area, often involving fractions, ensures a smooth renovation process.
4. Art and Design:
Artists and designers frequently work with fractions to achieve the right proportions and compositions in their creations. Fractions help them accurately divide canvases, mix colors, and achieve desired visual effects.
5. Sewing and Crafting:
When sewing clothes or creating crafts, fractions come into play for measuring fabric, cutting patterns, and ensuring precise stitches. Accurate fractions are essential for a well-fitting garment or a neatly crafted item.
Conclusion
So, that’s it about how to convert a decimal to fraction! It is an essential skill that empowers individuals to work with numbers in various contexts. By breaking down the process into manageable steps and practicing with examples, anyone can become proficient at this conversion.
In the realm of technology and science, this process mirrors the functioning of sensors – devices that play a vital role in gathering data from the physical world and converting it into measurable and understandable signals.
Speaking of sensors, WIN SOURCE‘s commitment to quality and accuracy aligns with the principles underlying the function of sensors. Just as sensors ensure precise data collection by counting, measuring, and calibrating, WIN SOURCE employs rigorous quality control measures to provide customers with components that meet stringent standards. Don’t forget to visit our store.

COMMENTS